What is 6÷2(1+2) = ?
The problem often generates debate and has millions of comments on Facebook, Twitter, YouTube and other social media sites.
The order of operations
The expression can be simplified by the order of operations, often remembered by the acronyms PEMDAS/BODMAS.
First evaluate Parentheses/Brackets, then evaluate Exponents/Orders, then evaluate Multiplication-Division, and finally evaluate Addition-Subtraction.
Everyone is in agreement about the first step: simplify the addition inside of the parentheses.
6÷2(1+2)
= 6÷2(3)
= 6÷2(3)
This is where the debate starts.
The answer is 9
If you type 6÷2(3) into a calculator, Google or WolframAlpha, the input has to be parsed and then computed. All of these will first convert the parentheses into an implied multiplication. The expression becomes the following.
6÷2(3)
= 6÷2×3
= 6÷2×3
According to the order of operations, division and multiplication have the same precedence, so the correct order is to evaluate from left to right. First take 6 and divide it by 2, and then multiply by 3.
6÷2×3
= 3×3
= 9
= 3×3
= 9
This gets to the correct answer of 9.
This is without argument the correct answer of how to evaluate this expression according to current usage.
Some people have a different interpretation. And while it’s not the correct answer today, it would have been regarded as the correct answer 100 years ago.
The other result of 1
Suppose it was 1917 and you saw 6÷2(3) in a textbook. What would you think the author was trying to write?
Historically the symbol ÷ was used to mean you should divide by the entire product on the right of the symbol (see longer explanation below).
Under that interpretation:
6÷2(3)
= 6÷(2(3))
(Important: this is outdated usage!)
= 6÷(2(3))
(Important: this is outdated usage!)
From this stage, the rest of the calculation works by the order of operations. First we evaluate the multiplication inside the parentheses. So we multiply 2 by 3 to get 6. And then we divide 6 by 6.
6÷(2(3))
= 6÷6
= 1
= 6÷6
= 1
This gives the result of 1. This is not the correct answer; rather it is what someone might have interpreted the expression according to old usage.


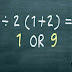


No comments:
Post a Comment